J. Nonl. Evol. Equ. Appl. 2022 (2), pp. 25-57, published on July 6, 2022: | Journal of Nonlinear Evolution Equations and Applications

Renormalized solutions for nonlinear elliptic system with variable exponents and singular coefficient and with diffuse measure data

Laboratoire MISI, FST Settat, Université Hassan 1, 26000 Settat, Morocco

Faculté d'économie et de gestion. Université Hassan 1, B.P. 764. Settat. Morocco.

Received on June 27, 2021
Accepted on February 16, 2022

Communicated by Ti-Jun Xiao

Abstract. In this paper we prove the existence of a renormalized solution for nonlinear elliptic system of the type: 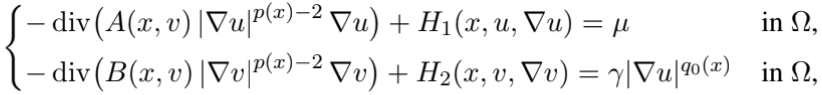where Ω is a bounded open subset of R^N, N ≥ 2, 2-1/N i(x, s, ξ) are Carathéodory functions. The function B(x, s) blows up (uniformly with respect to x) as s → m^- (with m > 0), and γ is a positive constant and q₀(x) ∈ [ 1, N(p(x)-1)/(N-1) ).
Keywords:	Nonlinear elliptic system. Singular coefficient. Variable exponents. Renormalized solutions.
2010 Mathematics Subject Classification: 47A15; Secondary 46A32; 47D20.